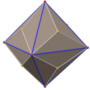
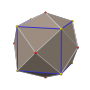
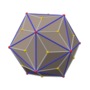
五角二十四面体
 (按这里观看旋转模型) | ||||
| 类别 | 卡塔兰立体 | |||
|---|---|---|---|---|
| 对偶多面体 | 扭棱立方体 | |||
| 识别 | ||||
| 鲍尔斯缩写 | pedid | |||
| 数学表示法 | ||||
| 考克斯特符号 | ||||
| 性质 | ||||
| 面 | 24 | |||
| 边 | 60 | |||
| 顶点 | 38 | |||
| 欧拉特征数 | F=24, E=60, V=38 (χ=2) | |||
| 二面角 | 136° 18' 33' | |||
| 组成与布局 | ||||
| 面的种类 | V3.3.3.3.4 不等边五边形 | |||
| 对称性 | ||||
| 对称群 | O, ½BC3, [4,3]+, 432 | |||
| 旋转对称群 | O, [4,3]+, (432) | |||
| 特性 | ||||
| 凸、面可递 | ||||
| 图像 | ||||
| ||||
在几何学中,五角二十四面体是一种卡塔兰多面体[1],由24个全等的不等边五边形组成,其对偶多面体为扭棱立方体[2],共有24个面、60个边和38个顶点[3]。
在矿物学中,这种形状又称为五角三八面体、螺旋二十四面体(gyroid)[4][5][6]、五角偏方三八面体或偏菱五角二十四面体[7],部分的矿石可以结晶成这种形状[8],例如赤铜矿——化学成份为氧化亚铜(Cu2O)的氧化物矿物可以结晶成五角二十四面体[9]。
性质
[编辑]五角二十四面体是一个手性多面体[10],也就是说,该多面体镜射之后会跟原本的型形状不同,无法借由旋转半周再回到原本的形状[11][12][13]。这两种形式互为镜像(或“对映体”),又称为手性镜像,且其面、顶点、边数皆相同,共有24个面、60个边、38个顶点[3]。
 五角二十四面体的旋转透视图 |
 五角二十四面体的另一个手性镜像的旋转透视图 |
五角二十四面体的对偶多面体为扭棱立方体,换句话说即这个多面体的顶点可以对应到扭棱立方体每个面的几何中心、扭棱立方体的每个顶点可以对应到五角二十四面体的几何中心。[14]
面的组成
[编辑]
五角二十四面体由24个全等的具有镜像对称性之不等边五边形组成[13][12]。这种不等边五边形有两种边长,有三个边为短边(下图中以b表示)、两个边为长边(下图中以a表示)。长边的边长为短边的一半再加上短边的三波那契常数倍[15],即:
- 短边长边
其中,为三波那契常数,即:
这个数为的实根[16]。
这个不等边五边形两个长边相邻,其夹角为二减去三波那契常数的反馀弦值(约为80.75度);其馀4个角皆为二分之一减去一半的三波那契常数之反馀弦值(约为114.81度)[15]。
若对应的对偶多面体——扭棱立方体边长为单位长,则相应的五角二十四面体面的短边边长为[13][12]:
体积与表面积
[编辑]若对应的对偶多面体——扭棱立方体边长为单位长,则相应的五角二十四面体的体积与表面积为[10]:
而根据相应的边长关系[13][12],可以得到以边长表示的体积与表面积:
正交投影
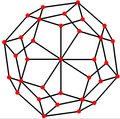
[编辑]五角二十四面体有三种具有特殊对称性的正交投影,分别是以度为三的顶点为中心、以度为四的顶点为中心以及以与侧边中点为中心的正交投影。前两者对称性对分别应于A2和B2的考克斯特平面[17][18]。
| 投影位置 | 度为三的顶点 | 度为四的顶点 | 侧边中点 |
|---|---|---|---|
| 投影对称性 | [3] | [4]+ | [2] |
| 图像 | 
|
|

|
| 对偶多面体 | 
|

|
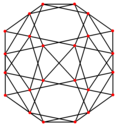
|
变体
[编辑]五角二十四面体有另外一种同样所有面全等的变体。这种变体具有八面体群的对称性,且具有3种不同的边长。这种变体可以透过在扭棱立方体的6个正方形与8个三角形的面上加上角锥至与邻面共面来构造[19]。
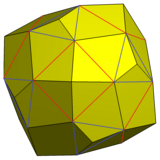 扭棱立方体的面上加上角锥至与邻面共面 |
 五角二十四面体变体 |
 该变体地展开图 |
相关多面体及镶嵌
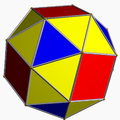
[编辑]五角二十四面体的拓朴结构属于(432)的旋转对称性[20],其他同为(n32)旋转对称性的几何结构有:
| 扭棱镶嵌对称性 n32 的变种: 3.3.3.3.n | ||||||||
|---|---|---|---|---|---|---|---|---|
| 对称性 n32 |
球面镶嵌 | 欧氏镶嵌 | 紧凑双曲 | 仿紧双曲 | ||||
| 232 | 332 | 432 | 532 | 632 | 732 | 832 | ∞32 | |
| 考克斯特记号 | ||||||||
| 扭棱图 | 
|

|

|

|

|

|

|

|
| 顶点图 | 3.3.3.3.2 | 3.3.3.3.3 | 3.3.3.3.4 | 3.3.3.3.5 | 3.3.3.3.6 | 3.3.3.3.7 | 3.3.3.3.8 | 3.3.3.3.∞ |
| 扭棱对偶 | 
|
|

|

|

|

|

| |
| 顶点布局 | V3.3.3.3.2 | V3.3.3.3.3 | V3.3.3.3.4 | V3.3.3.3.5 | V3.3.3.3.6 | V3.3.3.3.7 | V3.3.3.3.8 | V3.3.3.3.∞ |
关于的拓朴结构属于(432)的旋转对称性的五角二十四面体[20],亦可以从(4n2)旋转对称性进行比较。这些相关几何结构包括:
| 扭棱镶嵌对称性 4n2 的变种: 3.3.4.3.n | ||||||||
|---|---|---|---|---|---|---|---|---|
| 对称性 4n2 |
球面镶嵌 | 欧氏镶嵌 | 紧凑双曲 | 仿紧双曲 | ||||
| 242 | 342 | 442 | 542 | 642 | 742 | 842 | ∞42 | |
| 扭棱图 | 
|

|

|

|

|

|

|

|
| 顶点布局 | 3.3.4.3.2 | 3.3.4.3.3 | 3.3.4.3.4 | 3.3.4.3.5 | 3.3.4.3.6 | 3.3.4.3.7 | 3.3.4.3.8 | 3.3.4.3.∞ |
| 扭棱对偶 | 
|

|

|

|
||||
| 顶点布局 | V3.3.4.3.2 | V3.3.4.3.3 | V3.3.4.3.4 | V3.3.4.3.5 | V3.3.4.3.6 | V3.3.4.3.7 | V3.3.4.3.8 | V3.3.4.3.∞ |
五角二十四面体是立方体经过扭棱变换后的对偶多面体[10],其他也是由立方体透过康威变换得到的多面体有:
| 对称性: [4,3], (*432) | [4,3]+ (432) |
[1+,4,3] = [3,3] (*332) |
[3+,4] (3*2) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| {4,3} | t{4,3} | r{4,3} r{31,1} |
t{3,4} t{31,1} |
{3,4} {31,1} |
rr{4,3} s2{3,4} |
tr{4,3} | c{4,3} | sr{4,3} | h{4,3} {3,3} |
h2{4,3} t{3,3} |
s{3,4} s{31,1} |
= |
= |
= |
|||||||||
| 对偶多面体 | |||||||||||
| V43 | V3.82 | V(3.4)2 | V4.62 | V34 | V3.43 | V4.6.8 | V4.62/63 | V34.4 | V33 | V3.62 | V35 |
五角二十四面体图
[编辑]| 五角二十四面体图 | |
|---|---|
 | |
| 度分布 | 3 (32个) 4 (6个) |
| 顶点 | 38 |
| 边 | 60 |
| 半径 | 6 |
| 直径 | 7 |
| 围长 | 5 |
| 自同构群 | 24 |
| 色数 | 3 |
| 对偶图 | 扭棱立方体图 |
| 属性 | 哈密顿、平面图 |
在图论的数学领域中,与五角二十四面体相关的图为五角二十四面体图,是五角二十四面体之边与顶点的图,同时也是拓朴结构与五角二十四面体等架的图论对象,由38个节点和60条边组成[21],是一个哈密顿图[22]。
性质
[编辑]五角二十四面体图有60条边和38个顶点,其中度为3的顶点有32个;度为4的顶点有6个。这个图的直径是7,半径是6[22],其中半径代表图中所有顶点偏心率的最小值、直径代表代表图中所有顶点偏心率的最大值、偏心率为某顶点和离其最远点的距离[23]。换句话说五角二十四面体图在不考虑循环路径下顶点间最大距离只少相距6个顶点,最长距离不超过7个顶点[22]。五角二十四面体图的围长为5,即在这个图内最小的循环路径为5个顶点[22]。
 五角二十四面体的平行投影是一种五角二十四面体图 |
 以类似施莱格尔图的方式呈现的五角二十四面体图 |
 五角二十四面体图的另一种表示法 |
参见
[编辑]参考文献
[编辑]- Williams, Robert. The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. 1979. ISBN 0-486-23729-X. (Section 3-9)
- ^ The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ISBN 978-1-56881-220-5 [1] (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, page 287, pentagonal icosikaitetrahedron)
- ^ Wenninger, Magnus, Dual Models, Cambridge University Press, 1983, ISBN 978-0-521-54325-5, MR730208 (The thirteen semiregular convex polyhedra and their duals, Page 28, Pentagonal icositetrahedron)
- ^ 3.0 3.1 Pentagonal Icositetrahedron. polyhedra.org. [2020-08-01]. (原始内容存档于2008-07-14).
- ^ Promorphology of Crystals I. www.metafysica.nl. [2020-08-03]. (原始内容存档于2020-08-03).
- ^ Stephen A. Nelson. Crystal Form, Zones, Crystal Habit. 2011-01-11 [2020-08-03]. (原始内容存档于2013-09-01).
- ^ 五角三八面體;螺旋二十四面體 gyroid. 国家教育研究院. [2020-08-01]. (原始内容存档于2020-08-24).
- ^ 偏菱五角二十四面體 pentagonal icositetrahedron. 国家教育研究院. [2020-08-01]. (原始内容存档于2020-08-24).
- ^ 中川宏. 貫入双晶模型の製作 (PDF). [2018-08-30]. (原始内容 (PDF)存档于2018-08-31).
- ^ Hugo Steinhaus. Mathematical Snapshots (Dover Recreational Math). Dover Publications. 2011年12月28日: pp. 207, 209. ISBN 978-0486409146.
- ^ 10.0 10.1 10.2 Weisstein, Eric W. (编). Pentagonal Icositetrahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Coxeter, H. S. M., Kaleidoscopes: Selected Writings, John Wiley and Sons: 282, 1995, ISBN 9780471010036.
- ^ 12.0 12.1 12.2 12.3 12.4 Catalan Solids: Pentagonal Icositetrahedron (dextro). dmccooey.com. [2020-08-01]. (原始内容存档于2020-08-24).
- ^ 13.0 13.1 13.2 13.3 13.4 Catalan Solids: Pentagonal Icositetrahedron (laevo). dmccooey.com. [2020-08-01]. (原始内容存档于2020-08-24).
- ^ Holden, A. Shapes, Space, and Symmetry. Dover Books on Mathematics. Dover Publications. 1991: p.55. ISBN 9780486268514. LCCN 91020471.
- ^ 15.0 15.1 Pentagonal icositetrahedron. fillygons.ch. [2020-08-01]. (原始内容存档于2020-08-24).
- ^ Sloane, N.J.A. (编). Sequence A058265 (Decimal expansion of the tribonacci constant t, the real root of ). The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ 约翰·史坦布里奇. Coxeter Planes. math.lsa.umich.edu. [2020-08-01]. (原始内容存档于2018-02-10) (英语).
- ^ 约翰·史坦布里奇. More Coxeter Planes. math.lsa.umich.edu. [2020-08-01]. (原始内容存档于2017-08-21) (英语).
- ^ Koca, Nazife and Koca, Mehmet. Regular and Irregular Chiral Polyhedra from Coxeter Diagrams via Quaternions. Symmetry. 2017-08, 9: 148. doi:10.3390/sym9080148.
- ^ 20.0 20.1 Livio Zefiro, Maria Rosa Ardigo. What Became of the Controversial Fourteenth Archimedean Solid, the Pseudo Rhomb-Cuboctahedron?. Dip.Te.Ris, Universita' di Genova, Italy. [2020-08-24]. (原始内容存档于2020-07-31).
- ^ Hao, Jianqiang and Gong, Yunzhan and Sun, Jianzhi and Tan, Li. Use the K-Neighborhood Subgraphs to Compute Canonical Labelings of Graphs. Mathematics (Multidisciplinary Digital Publishing Institute). 2019, 7 (8): 690.
- ^ 22.0 22.1 22.2 22.3 22.4 Weisstein, Eric W. (编). Pentagonal Icositetrahedral Graph. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Chartrand G., Johns G., Oellermann O.R. On Peripheral Vertices in Graphs. Bodendiek R., Henn R. (编). Topics in Combinatorics and Graph Theory. Physica-Verlag HD. 1990.







![{\displaystyle {\frac {1+{\sqrt[{3}]{19+3{\sqrt {33}}}}+{\sqrt[{3}]{19-3{\sqrt {33}}}}}{3}}={\frac {1+4\cosh \left({\frac {1}{3}}\cosh ^{-1}\left(2+{\frac {3}{8}}\right)\right)}{3}}\approx 1.839286755214161,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/35d67dcf371181e0d9563c8328e2642102ddcce3)




![{\displaystyle b={\frac {\sqrt {6\left(4-{\sqrt[{3}]{2\left(13+3{\sqrt {33}}\right)}}-{\sqrt[{3}]{2\left(13-3{\sqrt {33}}\right)}}\right)}}{6}}\approx 0.593465355971987310502}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5b65438f953d1db2cfd40bb40bf2a66ca3b9a971)
![{\displaystyle a={\frac {\sqrt {3\left(4+{\sqrt[{3}]{19+3{\sqrt {33}}}}+{\sqrt[{3}]{19-3{\sqrt {33}}}}\right)}}{6}}\approx 0.8425091624448604672504}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1129f13464cb168fe4aa39b33de7613e3983d9df)