本条目中,向量 与标量 分别用粗体 与斜体 显示。例如,位置向量通常用
r
{\displaystyle \mathbf {r} \,\!}
r
{\displaystyle r\,\!}
在电磁学 里,电荷密度 是一种度量 ,用以描述空间中连续电荷 的分布状况。依据讨论电磁模型的维度而定,电荷密度可以是线电荷密度 、面电荷密度 或体电荷密度 。
假设电荷分布于一条曲线或一根直棒子,则其线电荷密度是每单位长度的电荷密度,单位为库仑 /公尺 (coulomb/meter) 。假设电荷分布于一个平面或一个物体的表面,则其面电荷密度是每单位面积的电荷密度,单位为库仑/公尺2 。假设电荷分布于一个三维空间的某区域或物体内部,则其体电荷密度是每单位体积的电荷密度,单位为库仑/公尺3 。
由于在大自然里,有两种电荷,正电荷 和负电荷 ,所以,电荷密度可能会是负值。电荷密度也可能会跟位置有关。特别注意,不要将电荷密度与电荷载子密度 (charge carrier density ) 搞混了。
电荷密度与电荷载子 的体积有关。例如,由于锂 阳离子 的半径比较小,它的体电荷密度大于钠 阳离子的体电荷密度。
假设,一个体积为
V
{\displaystyle V}
载电体 ,其电荷密度
ρ
0
{\displaystyle \rho _{0}}
Q
{\displaystyle Q}
Q
=
ρ
0
V
{\displaystyle Q=\rho _{0}V}
假设,在某一区域内有
N
{\displaystyle N}
点电荷 ,像电子 。那么,电荷密度可以用狄拉克δ函数 来表达为
ρ
(
r
)
=
∑
i
=
1
N
q
i
δ
(
r
−
r
i
)
{\displaystyle \rho (\mathbf {r} )=\sum _{i=1}^{N}\ q_{i}\delta (\mathbf {r} -\mathbf {r} _{i})}
其中,
r
{\displaystyle \mathbf {r} }
q
i
{\displaystyle q_{i}}
r
i
{\displaystyle \mathbf {r} _{i}}
i
{\displaystyle i}
电量 。
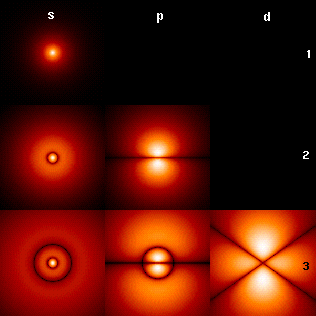
氢原子 的电子机率密度绘图。横排显示不同的角量子数 (l) ,竖排显示不同的能级 (n) 。这也是氢原子的负电荷密度图。氢原子的质子 的中心有一个正电性的质子 。在量子力学 里,类氢原子 的中心有一个正电性的原子核 ,环绕著原子核四周的一个电子的轨域,其电荷密度可以用波函数
ψ
(
r
)
{\displaystyle \psi (\mathbf {r} )}
[ 1]
ρ
(
r
)
=
q
⋅
|
ψ
(
r
)
|
2
{\displaystyle \rho (\mathbf {r} )=q\cdot |\psi (\mathbf {r} )|^{2}}
其中,
q
{\displaystyle q}
注意到
|
ψ
(
r
)
|
2
{\displaystyle |\psi (\mathbf {r} )|^{2}}
机率 。经过归一化 ,在全部空间找到电子的机率是
∫
a
l
l
s
p
a
c
e
|
ψ
(
r
)
|
2
d
3
r
=
1
{\displaystyle \int _{all\ space}|\psi (\mathbf {r} )|^{2}\mathrm {d} ^{3}{r}=1}
例如,氢原子 的波函数
ψ
n
l
m
(
r
)
{\displaystyle \psi _{nlm}(\mathbf {r} )}
ψ
n
l
m
(
r
)
=
R
n
l
(
r
)
Y
l
m
(
θ
,
ϕ
)
{\displaystyle \psi _{nlm}(\mathbf {r} )=R_{nl}(r)Y_{l}^{m}(\theta ,\,\phi )}
其中,
R
n
l
{\displaystyle R_{nl}}
Y
l
m
(
θ
,
ϕ
)
{\displaystyle Y_{l}^{m}(\theta ,\,\phi )}
球谐函数 ,
n
{\displaystyle n}
主量子数 ,
l
{\displaystyle l}
角量子数 ,
m
{\displaystyle m}
磁量子数 。
从相对论 的角度来论述,导线 的长度与观察者的移动速度有关,所以电荷密度是一种相对论性观念。安东尼·法兰碁 (Anthony French )在他的著作中表明[ 2] 载流导线 。。使用闵可夫斯基图 ,法兰碁阐明,一条中性的载流导线,对于处于移动参考系的观察者而言,为什么会貌似载有净电荷密度。通过时空 坐标,研究电磁现象 的领域称为相对论性电磁学 (relativistic electromagnetism )。
电荷密度与电流密度 之间的关系式为:
∂
ρ
(
r
,
t
)
∂
t
+
∇
⋅
J
(
r
,
t
)
=
0
{\displaystyle {\frac {\partial \rho (\mathbf {r} ,\,t)}{\partial t}}+{\boldsymbol {\nabla }}\cdot \mathbf {J} (\mathbf {r} ,\,t)=0}
其中,
r
{\displaystyle \mathbf {r} }
t
{\displaystyle t}
J
{\displaystyle \mathbf {J} }
在电磁理论 里,从马克士威方程组 ,可以推导出电荷守恒的连续方程式 。根据加入位移电流 项目后的安培定律 [ 3]
∇
×
B
=
μ
0
J
+
μ
0
ϵ
0
∂
E
∂
t
{\displaystyle \nabla \times \mathbf {B} =\mu _{0}\mathbf {J} +\mu _{0}\epsilon _{0}{\frac {\partial \mathbf {E} }{\partial t}}}
其中,
B
{\displaystyle \mathbf {B} }
E
{\displaystyle \mathbf {E} }
μ
0
{\displaystyle \mu _{0}}
磁常数 ,
ϵ
0
{\displaystyle \epsilon _{0}}
电常数 。
取散度 于方程式的两边:
∇
⋅
(
∇
×
B
)
=
μ
0
∇
⋅
J
+
μ
0
ϵ
0
∂
∂
t
(
∇
⋅
E
)
{\displaystyle \nabla \cdot (\nabla \times \mathbf {B} )=\mu _{0}\nabla \cdot \mathbf {J} +\mu _{0}\epsilon _{0}{\frac {\partial }{\partial t}}(\nabla \cdot \mathbf {E} )}
由于旋度 的散度等于零,再根据高斯定律 ,可以得到想要的关系式
0
=
∇
⋅
J
+
ϵ
0
∂
∂
t
(
∇
⋅
E
)
=
∇
⋅
J
+
∂
ρ
∂
t
{\displaystyle 0=\nabla \cdot \mathbf {J} +\epsilon _{0}{\frac {\partial }{\partial t}}(\nabla \cdot \mathbf {E} )=\nabla \cdot \mathbf {J} +{\frac {\partial \rho }{\partial t}}}
换另外一种比较直觉的推导方法。流入某体积
V
{\displaystyle \mathbb {V} }
I
=
−
∮
S
J
⋅
d
2
r
{\displaystyle I=-\oint _{\mathbb {S} }\mathbf {J} \cdot \mathrm {d} ^{2}\mathbf {r} }
其中,
I
{\displaystyle I}
S
{\displaystyle \mathbb {S} }
V
{\displaystyle \mathbb {V} }
d
r
2
{\displaystyle \mathrm {d} \mathbf {r} ^{2}}
S
{\displaystyle \mathbb {S} }
应用散度定理 ,将这方程式写为
I
=
−
∫
V
∇
⋅
J
d
3
r
{\displaystyle I=-\int _{\mathbb {V} }\nabla \cdot \mathbf {J} \ \mathrm {d} ^{3}r}
总电荷量
Q
{\displaystyle Q}
V
{\displaystyle \mathbb {V} }
ρ
{\displaystyle \rho }
Q
=
∫
V
ρ
d
3
r
{\displaystyle Q=\int _{\mathbb {V} }\rho \ \mathrm {d} ^{3}r}
电荷守恒要求,流入体积
V
{\displaystyle \mathbb {V} }
V
{\displaystyle \mathbb {V} }
Q
{\displaystyle Q}
d
Q
d
t
=
I
=
∫
V
∂
ρ
∂
t
d
3
r
{\displaystyle {\frac {\mathrm {d} Q}{\mathrm {d} t}}=I=\int _{\mathbb {V} }{\frac {\partial \rho }{\partial t}}\ \mathrm {d} ^{3}r}
所以,
∫
V
∂
ρ
∂
t
+
∇
⋅
J
d
3
r
=
0
{\displaystyle \int _{\mathbb {V} }{\frac {\partial \rho }{\partial t}}+\mathbf {\nabla } \cdot \mathbf {J} \ \mathrm {d} ^{3}r=0}
对于任意体积
V
{\displaystyle \mathbb {V} }
[ 4]
∂
ρ
∂
t
+
∇
⋅
J
=
0
{\displaystyle {\frac {\partial \rho }{\partial t}}+\nabla \cdot \mathbf {J} =0}
在一个体积区域
V
′
{\displaystyle \mathbb {V} '}
r
′
{\displaystyle \mathbf {r} '}
ρ
(
r
′
)
{\displaystyle \rho (\mathbf {r} ')}
r
{\displaystyle \mathbf {r} \!}
电势 为[ 3]
ϕ
(
r
)
=
1
4
π
ϵ
0
∫
V
′
ρ
(
r
′
)
|
r
−
r
′
|
d
3
r
′
{\displaystyle \phi (\mathbf {r} )={\frac {1}{4\pi \epsilon _{0}}}\int _{\mathbb {V} '}{\frac {\rho (\mathbf {r} ')}{|\mathbf {r} -\mathbf {r} '|}}\mathrm {d} ^{3}{r}'}
其中,
d
3
r
′
{\displaystyle \mathrm {d} ^{3}{r}'}
电场
E
{\displaystyle \mathbf {E} }
梯度 :
E
(
r
)
=
−
∇
ϕ
(
r
)
=
1
4
π
ϵ
0
∫
V
′
ρ
(
r
′
)
r
−
r
′
|
r
−
r
′
|
3
d
3
r
′
{\displaystyle \mathbf {E} (\mathbf {r} )=-{\boldsymbol {\nabla }}\phi (\mathbf {r} )={\frac {1}{4\pi \epsilon _{0}}}\int _{\mathbb {V} '}\rho (\mathbf {r} '){\frac {\mathbf {r} -\mathbf {r} '}{|\mathbf {r} -\mathbf {r} '|^{3}}}\mathrm {d} ^{3}{r}'}
应用向量关系式
∇
⋅
r
−
r
′
|
r
−
r
′
|
3
=
4
π
δ
(
r
−
r
′
)
{\displaystyle {\boldsymbol {\nabla }}\cdot {\frac {\mathbf {r} -\mathbf {r} '}{|\mathbf {r} -\mathbf {r} '|^{3}}}=4\pi \delta (\mathbf {r} -\mathbf {r} ')}
取散度于电场,
∇
⋅
E
(
r
)
=
−
∇
2
ϕ
(
r
)
=
1
4
π
ϵ
0
∫
V
′
ρ
(
r
′
)
4
π
δ
(
r
−
r
′
)
d
3
r
′
{\displaystyle {\boldsymbol {\nabla }}\cdot \mathbf {E} (\mathbf {r} )=-\nabla ^{2}\phi (\mathbf {r} )={\frac {1}{4\pi \epsilon _{0}}}\int _{\mathbb {V} '}\rho (\mathbf {r} ')4\pi \delta (\mathbf {r} -\mathbf {r} ')\mathrm {d} ^{3}{r}'}
可以得到高斯定律 的微分形式
∇
⋅
E
(
r
)
=
ρ
(
r
)
ϵ
0
{\displaystyle {\boldsymbol {\nabla }}\cdot \mathbf {E} (\mathbf {r} )={\frac {\rho (\mathbf {r} )}{\epsilon _{0}}}}
和帕松方程式
∇
2
ϕ
(
r
)
=
−
ρ
(
r
)
ϵ
0
{\displaystyle \nabla ^{2}\phi (\mathbf {r} )=-{\frac {\rho (\mathbf {r} )}{\epsilon _{0}}}}
^ Cao, Tian Yu, Conceptual developments of 20th century field theories reprint, illustrated, Cambridge University Press: pp. 146–147, 1998, ISBN 9780521634205 ^ A. French (1968) Special Relativity , chapter 8 Relativity and electricity, pp 229–65, W. W. Norton.
^ 3.0 3.1 Jackson, John David, Classical Electrodynamic 3rd., USA: John Wiley & Sons, Inc.: pp. 29–31, 237–239, 1999, ISBN 978-0-471-30932-1 ^ Griffiths, David J., Introduction to Electrodynamics (3rd ed.), Prentice Hall: pp. 213, 1998, ISBN 0-13-805326-X