狄默夫公式

複數平面上的立方根等於1.

狄默夫公式（英語：de Moivre's formula）是一個關於複數和三角函數的公式，命名自法國數學家亞伯拉罕·狄默夫（1667年－1754年）。其內容為對任意實數$x$和整數$n$，下列性質成立：

$(\cos(x)+i\sin(x))^{n}=\cos(nx)+i\sin(nx)$

其中$i$是虛數單位（$i^{2}=-1$）。值得注意的是，儘管本公式以狄默夫本人命名，他從未直接地將其發表過。為了方便起見，我們常常將$\cos x+i\sin x$合併為另一個三角函數cis(x)，也就是說：

$\operatorname {cis} ^{n}(x)=\operatorname {cis} (nx)$

在操作上，我們常常限制$x$屬於實數，這樣一來就可藉由比較虛部與實部的方式把$\cos(nx)$和$\sin(nx)$變化為$\cos x$和$\sin x$的形式。另外，儘管狄默夫公式限制$n$須為整數，但倘若適當推廣本公式，便可將$n$拓展到非整數的領域。

證明

（證明的思路是用數學歸納法證明正整數的情形，並推廣到負整數。）

令$P(n)=(\cos \theta +i\sin \theta )^{n}=\cos(n\theta )+i\sin(n\theta ),n\in \mathbb {N}$

（1）當$n=0$時，顯然成立。

（2）當$n=1$時：

左式 $=(\cos \theta +i\sin \theta )^{1}=\cos \theta +i\sin \theta =\cos(1\cdot \theta )+i\sin(1\cdot \theta )=$ 右式

因此，$P(1)$成立。

（3）當$n>1$時：

假設$P(k)$成立，即$(\cos \theta +i\sin \theta )^{k}=\cos(k\theta )+i\sin(k\theta )$

當$n=k+1$時：

${\begin{aligned}(\cos \theta +i\sin \theta )^{k+1}&=(\cos \theta +i\sin \theta )^{k}\cdot (\cos \theta +i\sin \theta )\\&=(\cos k\theta +i\sin k\theta )\cdot (\cos \theta +i\sin \theta )\\&=(\cos k\theta \cdot \cos \theta +i\sin k\theta \cdot i\sin \theta )+(\cos k\theta \cdot i\sin \theta +i\sin k\theta \cdot \cos \theta )\\&=(\cos k\theta \cdot \cos \theta -\sin k\theta \cdot \sin \theta )+i(\cos k\theta \cdot \sin \theta +\sin k\theta \cdot \cos \theta )\\&\ {\overset {1}{=}}\cos(k\theta +\theta )+i\sin(k\theta +\theta )\\&\ =\cos[(k+1)\theta ]+i\sin[(k+1)\theta ]\\\end{aligned}}$

等號1處使用和角公式。

因此，$P(k+1)$也成立。

綜上所述，根據數學歸納法，$\forall n\in \mathbb {N}$，$P(n)$成立。

另外，由恆等式：

$(\cos(n\theta )+i\sin(n\theta ))\cdot (\cos(-n\theta )+i\sin(-n\theta ))=1$

可知，公式對於負整數情況也成立。

證畢。

檢驗

最簡單的方法是應用歐拉公式。

由於$e^{ix}=\cos x+i\sin x\,$

所以${\color {Green}(\cos x+i\sin x)^{n}}=(e^{ix})^{n}=e^{inx}=e^{i(nx)}={\color {Green}\cos(nx)+i\sin(nx)}$

用狄默夫公式求根

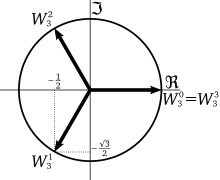
此定理可用來求單位複數的 $n$ 次方根。設 $|z|=1$，表為

$z=\cos \theta +i\sin \theta$

若 $w^{n}=z$，則 $w$ 也可以表成：

$w=\cos \phi +i\sin \phi$

按照狄默夫公式：

$w^{n}=(\cos \phi +i\sin \phi )^{n}=\cos n\phi +i\sin n\phi =\cos \theta +i\sin \theta =z$

於是得到

$n\phi =\theta +2k\pi$（其中 $k\in \mathbb {Z}$）

也就是：

$\phi ={\dfrac {\theta +2k\pi }{n}}$

當 $k$ 取 $0,1,\ldots ,n-1$，我們得到 $n$ 個不同的根：

$w=\cos({\dfrac {\theta +2k\pi }{n}})+i\sin({\dfrac {\theta +2k\pi }{n}}),k=0,1,\ldots ,n-1$