用戶:Sfc9982/地月轉移

地月轉移(英語:trans-lunar injection (TLI))是月球探測器從地球出發,通過推進器加速,進而脫離地球引力,最終被月球引力捕獲的過程。
歷史
[編輯]
GRAIL-A · 月球 · 地球

地球 · 月球 · Chandrayaan-2
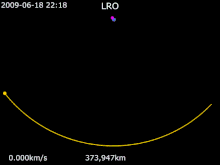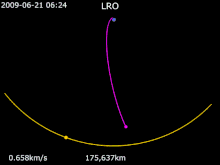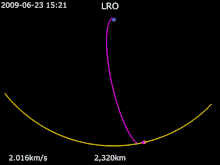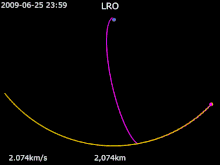
月球勘測軌道飛行器(LRO) · 地球 · 月球
蘇聯於1959年1月2日發射的月球1號是第一個嘗試地月轉移的探測器,月球1號原本計劃直接撞擊月球,但是地面控制系統的故障導致火箭的點燃時間出現誤差,導致探測器偏離計劃路線。最終探測器錯失了被月球捕獲的機會,以3倍原軌道半徑的距離與月球擦肩而過。月球1號在掠過月球表面後,隨即離開地球軌道,進入了日心軌道。[1][2] 月球2號在1959年9月12日更準確地執行了相同的機動,最終在2天後成功撞擊月球表面。[3] 蘇聯航天部門在1959至1976年間成功重複了多次類似的太空任務:22次「月球」任務、5次「探測器」任務。[4]
美國在則在1962年1月26日發射了其第一個月球撞擊探測器,徘徊者3號,但最終未能抵達月球。但此後的徘徊者4號於1962年4月23日成功完成了此項使命。[5] 在1962至1973年間美國重複了其他類似的27次太空任務,包括5次成功的勘測者計劃軟着陸器,5次月球軌道計劃探測太空船,[6]:166 和9次阿波羅任務,搭載人類首次登陸月球。
第一次載人地月轉移任務是1968年12月21日發射的阿波羅8號,使其組員成為第一批離開近地軌道的人類。[7]
在阿波羅計劃的月球任務中,地月轉移由火星5號火箭S-IVB的第三級可重啟J-2發動機完成。 這種特別的地月轉移推進持續了大約350秒,為飛行器提供了3.05 - 3.25 km/s的速度變化,此時飛行器正在以相對於地球10.4 km/s的速度前進。[8] 在傍晚天色的映襯下,阿波羅8號的地月轉移過程可以從夏威夷瓦基基島南部被清晰地看到,飛行器被人們拍了照,並在第二天被紙面報道。[9] 同樣地在1969年,阿波羅10號在黃昏中的地月轉移過程在澳大利亞的吉朗卡列依然可見。[10] 目擊者將場景描述為:一對車燈從霧中的山尖後躍出,此時的飛行器像極了一個拖着綠色彗尾的彗星。[10]
1990年,日本發射了其第一個月球任務飛行器——飛天號,計劃飛掠月球並將羽衣號微型衛星送入月球軌道。 此後,它探索了一種新的低Delta-v地月轉移路徑,全程用時6個月(而阿波羅選擇的耗時為 3 天)。[11][6]:179
1994年,美國的克萊門汀號探測器為了展示其機體的輕量級技術,在進入月球軌道之前使用了一次為期 3 周的地月轉移和兩次中途地球飛越。[11][6]:185
1997年,亞洲3號通信衛星成為第一顆被月球引力捕獲的商業衛星,在發射失敗後,它以低Delta-v方式繞月兩次,以到達其所需的靜止軌道。在距離月球表面 6200公里的高度處飛過月球。[11][6]:203
2003年,歐空局的SMART-1技術示範衛星成為第一顆繞月飛行的歐洲衛星。在被發射到地球靜止轉移軌道(GTO)後,它使用太陽能離子發動機進行推進。由於其極低的Delta-v地月轉移機動性,該航天器花了13個多月才到達月球軌道,並又花了17個月才抵達其期望的軌道。[6]:229
中華人民共和國於2007年啟動了第一次月球太空任務,將嫦娥一號發射至月球軌道。衛星經理了數次推進才使其遠地點緩慢遠離地球,直到抵達月球附近。[6]:257
緊隨其後的2008年, 印度將其的Chandrayaan-1發射至地球靜止轉移軌道,然後和中國的飛行器一樣,使用數次緩慢的推進來提高遠地點到月球附近。[6]:259
以色列航太工業公司製造的月球軟着陸器創世紀號 在2019年使用了這種機動過程,但卻不幸在月球上墜毀。
在2011年,NASA的GRAIL衛星選擇了一條低Delta-v路線抵達月球,經過了地日拉格朗日L1點,整個過程歷時3個月。[6]:278
理論
[編輯]Typical lunar transfer trajectories approximate Hohmann transfers, although low-energy transfers have also been used in some cases, as with the Hiten probe.[12] For short duration missions without significant perturbations from sources outside the Earth-Moon system, a fast Hohmann transfer is typically more practical.
A spacecraft performs TLI to begin a lunar transfer from a low circular parking orbit around Earth. The large TLI burn, usually performed by a chemical rocket engine, increases the spacecraft's velocity, changing its orbit from a circular low Earth orbit to a highly eccentric orbit. As the spacecraft begins coasting on the lunar transfer arc, its trajectory approximates an elliptical orbit about the Earth with an apogee near to the radius of the Moon's orbit. The TLI burn is sized and timed to precisely target the Moon as it revolves around the Earth. The burn is timed so that the spacecraft nears apogee as the Moon approaches. Finally, the spacecraft enters the Moon's sphere of influence, making a hyperbolic lunar swingby.yyyyyyyyyyyyyyyyyyyyyyyyyyyyyyyy
自由返回
[編輯]
In some cases it is possible to design a TLI to target a free return trajectory, so that the spacecraft will loop around behind the Moon and return to Earth without need for further propulsive maneuvers.[13]
Such free return trajectories add a margin of safety to human spaceflight missions, since the spacecraft will return to Earth "for free" after the initial TLI burn. The Apollos 8, 10 and 11 began on a free return trajectory,[14] while the later missions used a functionally similar hybrid trajectory, in which a midway course correction is required to reach the moon.[15][16][17]
建模
[編輯]
橢圓軌道修正
[編輯]TLI targeting and lunar transfers are a specific application of the n body problem, which may be approximated in various ways. The simplest way to explore lunar transfer trajectories is by the method of patched conics. The spacecraft is assumed to accelerate only under classical 2 body dynamics, being dominated by the Earth until it reaches the Moon's sphere of influence. Motion in a patched-conic system is deterministic and simple to calculate, lending itself for rough mission design and "back of the envelope" studies.
有限圓軌道三體問題 (RC3B)
[編輯]More realistically, however, the spacecraft is subject to gravitational forces from many bodies. Gravitation from Earth and Moon dominate the spacecraft's acceleration, and since the spacecraft's own mass is negligible in comparison, the spacecraft's trajectory may be better approximated as a restricted three-body problem. This model is a closer approximation but lacks an analytic solution,[18] requiring numerical calculation.[19]
精度提高
[編輯]More detailed simulation involves modeling the Moon's true orbital motion; gravitation from other astronomical bodies; the non-uniformity of the Earth's and Moon's gravity; including solar radiation pressure; and so on. Propagating spacecraft motion in such a model is numerically intensive, but necessary for true mission accuracy
參考
[編輯]- ^ Luna 01. NASA.
- ^ Soviet Missions to the Moon. nssdc.gsfc.nasa.gov. [2021-07-25].
- ^ NASA - NSSDCA - Spacecraft - Details. nssdc.gsfc.nasa.gov.
- ^ Soviet Missions to the Moon. nssdc.gsfc.nasa.gov.
- ^ Ranger 4. NASA.
- ^ 6.0 6.1 6.2 6.3 6.4 6.5 6.6 6.7 Beyond Earth (PDF). NASA.
- ^ Mars, Kelli. 50 Years Ago: Apollo 8, You are Go for TLI!. NASA. December 20, 2018.
- ^ Apollo By the Numbers. NASA. (原始內容存檔於2004-11-18).
- ^ Independent Star News, Sunday, December 22, 1968. "The TLI firing was begun at PST while the craft was over Hawaii and it was reported there that the burn was visible from the ground."
- ^ 10.0 10.1 French, Francis; Colin Burgess. In the Shadow of the Moon. University of Nebraska Press. 2007: 372. ISBN 978-0-8032-1128-5.
- ^ 11.0 11.1 11.2 Alexander M. Jablonski1a; Kelly A. Ogden. A Review of Technical Requirements for Lunar Structures – Present Status (PDF). International Lunar Conference 2005. 2005.
- ^ Hiten. NASA.
- ^ Schwaninger, Arthur J. Trajectories in the Earth-Moon Space with Symmetrical Free Return Properties (PDF). Technical Note D-1833. Huntsville, Alabama: NASA / Marshall Space Flight Center. 1963.
- ^ Mansfield, Cheryl L. Apollo 10. NASA. May 18, 2017.
- ^ APOLLO 12. history.nasa.gov.
- ^ Ways to the Moon (PDF) (報告): 93.
- ^ Launch Windows Essay. history.nasa.gov.
- ^ Henri Poincaré, Les Méthodes Nouvelles de Mécanique Céleste, Paris, Gauthier-Villars et fils, 1892-99.
- ^ Victor Szebehely, Theory of Orbits, The Restricted Problem of Three Bodies, Yale University, Academic Press, 1967.
