引力势

提示：此条目的主题不是引力势能或引力场。

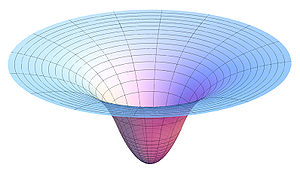
均匀球体内部和周围的引力势二维切片图。截面的拐点位于该球体的表面。

在经典力学中，一个位置上的引力势（英语：Gravitational potential）等于将每单位质量的物体从零势面移动到该位置所需的功（即此过程中转移给该单位质量的物体的能量）。引力势类似于电磁学中电势的概念，而质量可比拟为电荷在电磁学中扮演的角色。习惯上，引力势的零势面会取在无限远处。在这种约定下，任何有限距离处的引力势都小于零。

在数学上，引力势也称为牛顿势（英语：Newtonian potential），是势能理论的基础。势能理论也可以用于解释由均匀带电或极化的椭圆体产生的静电场和静磁场。

势能

主条目：引力势能

一个位置的引力势（$V$）等于每单位质量在该点拥有的势能（$U$）：

$V={\frac {U}{m}},$

式中$m$表示物体的质量。一个位置的引力势能等于在将物体从无限远处移动到该点的路径上，引力场所做的正功。若物体的质量等于1千克，那么该物体的势能的大小便会与引力势相等。

在某些情况下，可以假设引力场的强度与所在位置无关。此时上式可以被进一步化简。比方说，在接近地表附近的重力加速度$g$可以视为定值，因此不同位置间的势能差$\Delta U$能够与高度差$\Delta h$近似为简单的线性关系：

$\Delta U\approx mg\Delta h.$

数学形式

若令一质点的质量为$M$，则在与质点距离$\mathbf {r}$处的引力势$V$可被定义为：

$V(\mathbf {r} )=-{\frac {GM}{r}}$

推导

参见：保守向量场

牛顿万有引力定律指出：

$\mathbf {F} =-{\frac {GMm}{r^{2}}}\mathbf {\hat {r}}$

其中

• $\mathbf {F}$：质量$m$的质点受到的万有引力
• $G$：万有引力常数
• $m$：质点1的质量
• $M$：质点2的质量
• $r$：两个物体之间的距离
• $\mathbf {\hat {r}}$：由$M$指向$m$的单位向量

式中的负号使得$m$往$M$方向吸引，因此万有引力是吸引力。

而引力场$g$则描述了空间中任意位置上，每单位质量的质点所受到的万有引力：

$\mathbf {g} ={\frac {\mathbf {F} }{m}}=-G{\frac {M}{r^{2}}}\mathbf {\hat {r}}$

当我们去考虑在引力场中每单位质量的物体由外力移动一段距离$\mathbf {dl}$所需做的功$\mathbf {d} W$，由于功等于力与位移的内积，所以$d\mathbf {d} W=-\mathbf {g} \cdot \mathbf {dl}$，式中的负号表示外力所做的功与引力场所做的功相反。如果将物体从点$\mathbf {a}$移动到点$\mathbf {b}$，则$W$等于沿着该路径的线积分

$W=\int _{\mathbf {a} }^{\mathbf {b} }-\mathbf {g} \cdot \mathbf {dl}$

在球坐标系中$\mathbf {dl} =\mathbf {d} r\mathbf {\hat {r}} +r\mathbf {d} \theta {\boldsymbol {\hat {\theta }}}+r\sin {\theta }\mathbf {d} \phi {\boldsymbol {\hat {\phi }}}$，所以

$\mathbf {g} \cdot \mathbf {dl} =-{\frac {GM}{r^{2}}}\mathbf {d} r$

因此

${\begin{aligned}W&=\int _{\mathbf {a} }^{\mathbf {b} }-\mathbf {g} \cdot \mathbf {dl} \\&=\int _{\mathbf {a} }^{\mathbf {b} }{\frac {GM}{r^{2}}}\mathbf {d} r\\&=GM({\frac {1}{r_{a}}}-{\frac {1}{r_{b}}})\end{aligned}}$

其中，$r_{a}$是从原点到点$\mathbf {a}$的距离，$r_{b}$是从原点到点$\mathbf {b}$的距离。对于任何两条具有相同起点和终点的路径，上式的积分一定具有相同的值。既然线积分与路径无关，我们可以就定义一个函数$V(\mathbf {r} )$：

$V(\mathbf {r} )=-\int _{\mathcal {O}}^{\mathbf {r} }\mathbf {g} \cdot \mathbf {dl}$

$V(\mathbf {r} )$就称为引力势。只要预先设定一个标准参考点${\mathcal {O}}$，$V$的值就可以由$\mathbf {r}$来决定。

习惯上，我们将无限远处的引力势设为零。因此，在点$\mathbf {r}$的引力势$V$等于

$V(\mathbf {r} )=GM({\frac {1}{\infty }}-{\frac {1}{r}})=-{\frac {GM}{r}}$

此外，$W$可以用$V$重新写成：

${\begin{aligned}W&=\int _{\mathbf {a} }^{\mathbf {b} }-\mathbf {g} \cdot \mathbf {dl} \\&=-\int _{\mathbf {\mathcal {O}} }^{\mathbf {b} }\mathbf {g} \cdot \mathbf {dl} -\int _{\mathbf {a} }^{\mathbf {\mathcal {O}} }\mathbf {g} \cdot \mathbf {dl} \\&=-\int _{\mathbf {\mathcal {O}} }^{\mathbf {b} }\mathbf {g} \cdot \mathbf {dl} +\int _{\mathbf {\mathcal {O}} }^{\mathbf {a} }\mathbf {g} \cdot \mathbf {dl} \\&=V(\mathbf {b} )-V(\mathbf {a} )\end{aligned}}$

因此，在引力场中移动每单位质量的物体所需的功，等于两点之间引力势的差。如果想将物体移动到了离质点$M$更远的地方，则一定要做正功。上式也可以看做是将单位质量的物体从无限远处移到该点所需的功。

引力势的梯度

参见：梯度定理

由上述的计算得知，$\mathbf {a}$、$\mathbf {b}$两点之间引力势的差等于

$V(\mathbf {b} )-V(\mathbf {a} )=-\int _{\mathbf {a} }^{\mathbf {b} }\mathbf {g} \cdot \mathbf {dl}$

然而根据梯度定理（线积分基本定理），引力势的梯度$\mathbf {\nabla } V$沿曲线的积分，可用引力势在该曲线两端的值之差来计算：

$V(\mathbf {b} )-V(\mathbf {a} )=\int _{\mathbf {a} }^{\mathbf {b} }(\mathbf {\nabla } V)\cdot \mathbf {dl}$

所以

$\int _{\mathbf {a} }^{\mathbf {b} }(\mathbf {\nabla } V)\cdot \mathbf {dl} =-\int _{\mathbf {a} }^{\mathbf {b} }\mathbf {g} \cdot \mathbf {dl}$

由于对于任何点$\mathbf {a}$、$\mathbf {b}$都是如此，因此被积数必须相等：

$\mathbf {g} =-\mathbf {\nabla } V$

这是引力势的一个重要性质。

单位

在公制单位中，力的单位是牛顿，质量的单位是千克，所以引力场的单位是牛顿/千克而引力势的单位是牛顿公尺/千克，或焦耳/千克。

叠加

经典力学中，一个质量分布产生的引力势，等于各个点质量的引力势的叠加。如果一个质量分布由有限个点质量组成，点质量的位置为$\mathbf {r} _{1},...,\mathbf {r} _{n}$，质量为$m_{1},...,m_{n}$，那么其在点$\mathbf {r}$产生的引力势$V(\mathbf {r} )$等于

$V(\mathbf {r} )=\sum _{i=1}^{n}-{\frac {Gm_{i}}{|\mathbf {r} -\mathbf {r_{i}} |}}.$

如果在三维欧氏空间$\mathbf {R} ^{3}$上将质量分布以测度$\mathbf {d} m$给出，则引力势等于$-G/r$对$\mathbf {d} m$的卷积。 在理想的情况下，这等价于积分

$V(\mathbf {r} )=-\int _{\mathbf {R} ^{3}}{\frac {G}{|\mathbf {r} -\mathbf {r} \prime |}}\,\mathbf {d} m(\mathbf {r} \prime ),$

式中$|\mathbf {r} -\mathbf {r} \prime |$代表点$\mathbf {r}$与点$\mathbf {r} \prime$的距离。如果该质量分布在点$\mathbf {r}$的密度为$\rho (\mathbf {r} )$，那么$\mathbf {d} m$便等于密度$\rho (\mathbf {r} )$与单位体积 $\mathbf {d} \tau$的乘积：$\mathbf {d} m=\rho (\mathbf {r} )\mathbf {d} \tau$，而引力势就等于体积分

$V(\mathbf {r} )=-\int _{\mathbf {R} ^{3}}{\frac {G}{|\mathbf {r} -\mathbf {r} \prime |}}\,\rho (\mathbf {r} \prime )\mathbf {d} \tau (\mathbf {r} \prime ).$

泊松方程

主条目：引力场和高斯引力定律

如果有一个引力场$\mathbf {g}$由质量分布$\rho$产生，使用高斯定律（英语：Gauss's law for gravity）的微分形式可以获得

$\mathbf {\nabla } \cdot \mathbf {g} =-4\pi G\rho .$

由于$\mathbf {g} =-\mathbf {\nabla } V.$，带入高斯定律后可得到引力的泊松方程

${\nabla }^{2}V=4\pi G\rho .$

若密度处处为零，则上式便退化为拉普拉斯方程。泊松方程可以使用格林函数求解。

球形对称

主条目：壳层定理

根据壳层定理，若存在一个球形对称的质量分布，对对于处在分布外面的观察者而言，其行为就好像所有质量都集中在球心的个点质量，因此可以等效地作为点质量来处理。在地球表面，重力加速度g大约为9.8 m/s²，尽管该值随纬度和海拔高度略有变化（因为地球是扁球形，极点处的加速度大小略大于赤道处的加速度大小。）

在一个密度均匀的球体内，可以求出其引力势$V(r)$等于

$V(r)={\frac {2}{3}}\pi G\rho (r^{2}-3R^{2}),\qquad r\leq R.$

广义相对论

在广义相对论中，引力势被度量张量取代。当引力场的来源较弱并且移动速度比光速慢很多时，广义相对论就会简化为牛顿万有引力理论，且在一阶度规张量可表示为引力势的函数。

多极展开

主条目：球多极矩和多极展开

在计算空间中的引力势

$V(\mathbf {r} )=-\int _{\mathbf {R} ^{3}}{\frac {G}{|\mathbf {r} -\mathbf {r} \prime |}}\,\rho (\mathbf {r} \prime )\mathbf {d} \tau (\mathbf {r} \prime )$

时，牵涉到计算$|\mathbf {r} -\mathbf {r} \prime |$的倒数的积分，这个积分的难易度虽著质量分布$\rho$而异。为了将计算化简，这时候可以使用多极展开，将式子化为$1/r$的幂级数，让积分变得容易得多。做理论运算时，在允许误差范围内，时常可以只取多极展开几个最低阶的非零项，忽略其它剩下的、数值超小的项。

数值

主条目：地球引力

下表给出了关于来自地球，太阳和银河系的引力在不同位置上的引力势大小；换句话说，位于地球表面的物体需要60 MJ/kg的动能才能“脱离”地球的引力场，另外要有900 MJ/kg才能脱离太阳的引力场，而超过130 GJ/kg才能脱离银河系的引力场。引力势是逃离速度的平方的一半。

地点	地球引力的引力势	太阳引力的引力势	银河系引力的引力势
地球表面	60 MJ/kg	900 MJ/kg	≥ 130 GJ/kg
近地轨道	57 MJ/kg	900 MJ/kg	≥ 130 GJ/kg
旅行者1号 (距离地球170亿公里)	23 J/kg	8 MJ/kg	≥ 130 GJ/kg
距离地球 0.1 光年处	0.4 J/kg	140 kJ/kg	≥ 130 GJ/kg

参见

• 牛顿万有引力定律
• 高斯引力定律
• 地球引力
• 标准引力参数 (GM)
• 位势论
• 牛顿势
• 地球引力势模型
• 物理大地测量
• 勒让德多项式
• 三个变量的拉普拉斯方程的格林函数

参考资料

• Vladimirov, V. S., Equations of mathematical physics, Translated from the Russian by Au\mathbf{d}rey Littlewood. Edited by Alan Jeffrey. Pure and Applied Mathematics 3, New York: Marcel Dekker Inc., 1971, MR 0268497 .
• Wang, W. X. The potential for a homogeneous spheroid in a spheroidal coordinate system. I. At an exterior point. J. Phys. A: Math. Gen. 1988, 21: 4245-4250. Bibcode:1988JPhA...21.4245W. 
• Milon, T. A note on the potential of a homogenous ellipsoid in ellipsoidal coordinates. J. Phys. A: Math. Gen. 1990, 23: 581–584. doi:10.1088/0305-4470/23/4/027. 
• Rastall, Peter. Postprincipia: Gravitation for Physicists and Astronomers. World Scientific. 1991: 7ff. ISBN 981-02-0778-6. 
• Conway, John T. Exact solutions for the gravitational potential of a family of heterogeneous spheroids 316. 2000: 555=558. Bibcode:2000MNRAS.316..555C. doi:10.1046/j.1365-8711.2000.03524.x. 
• Cohl, H. S.; Tohline, J. E.; Rau, A. R. P. Developments in determining the grativational potential using toroidal functions 321. 2000: 363–372. Bibcode:2000AN....321..363C. doi:10.1002/1521-3994(200012)321:5/6<363::AID-ASNA363>3.0.CO;2-X. 
• Thornton, Stephen T.; Marion, Jerry B., Classical Dynamics of Particles and Systems 5th, Brooks Cole, 2003, ISBN 978-0-534-40896-1 .
• Zhu, Lupeia. Gravity and Earth's Density Structure. Department of Earth and Atmospheric Sciences. EAS-437 Earth Dynamics. Saint Louis University. California Institute of Technology. 1988 [2009-03-25]. 
• Charles D. Ghilani. The Gravity Field of the Earth. The Physics Fact Book. Penn State Surveying Engineering Program. 2006-11-28 [2009-03-25]. （原始内容存档于2011-07-18）. 
• Fukushima, Toshio. Prolate spheroidal harmonic expansion of gravitational field. Astrophys. J. 2014, 147 (6): 152. Bibcode:2014AJ....147..152F. doi:10.1088/0004-6256/147/6/152.