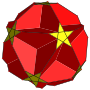
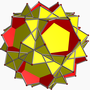
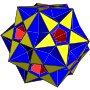
大截半二十面体
外观
 | |||
| 类别 | 星形均匀多面体 | ||
|---|---|---|---|
| 对偶多面体 | 大菱形三十面体 | ||
| 识别 | |||
| 名称 | 大截半二十面体 | ||
| 参考索引 | U54, C70, W94 | ||
| 鲍尔斯缩写 | gid | ||
| 数学表示法 | |||
| 考克斯特符号 | |||
| 施莱夫利符号 | |||
| 威佐夫符号 | 2 | 3 5/2 2 | 3 5/3 2 | 3/2 5/2 2 | 3/2 5/3 | ||
| 性质 | |||
| 面 | 32 | ||
| 边 | 60 | ||
| 顶点 | 30 | ||
| 欧拉特征数 | F=32, E=60, V=30 (χ=2) | ||
| 组成与布局 | |||
| 面的种类 | 20个正三角形 12个五角星 | ||
| 顶点图 | 3.5/2.3.5/2 | ||
| 顶点布局 | 20{3}+12{5/2} | ||
| 对称性 | |||
| 对称群 | Ih, [5,3], *532 | ||
| 特性 | |||
| 非凸 | |||
| 图像 | |||
| |||
在几何学中,大截半二十面体是一种非凸均匀多面体,属于星形多面体,其在非凸均匀多面体被编号为U54[1]、在温尼尔多面体模型被编号为W94[2]。其在施莱夫利符号中可以用r{3,5/2}表示,其为大星形十二面体和大二十面体的截半多面体。
性质
[编辑]大截半二十面体共有32个面、60条边和30个顶点[3],其30个面分别由20个正三角形和12个五角星组成[4][5],每个顶点都是2个三角形和2个五角星的公共顶点,在施莱夫利符号中可以用来表示[6]。
对偶多面体
[编辑]大截半二十面体的对偶多面体是大菱形三十面体,是一种等面多面体,所有面都由菱形组成,每个菱形被其他面相交的部分皆相同。但由于其不具有点可递的性质,也就是说,其并非所有顶角等角,因此不是均匀多面体。
作为凹多面体
[编辑]作为凹多面体,其可以视为由60个左右对称的五边形、12个正五边形和60个筝形组成,此种结构非常适合用于制作出大截半二十面体的纸模型[7]。
相关多面体
[编辑] 大截半二十面体 |
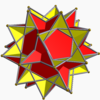 大十二面半十二面体 |
 大二十面半十二面体 |
 截半二十面体 (凸包) |
| 名称 | 大星形十二面体 | 截角大星形十二面体 | 大截半二十面体 | 截角大二十面体 | 大二十面体 |
|---|---|---|---|---|---|
| 考式 | |||||
| 图像 | 
|

|

|

|

|
对偶复合体
[编辑]大截半二十面体与其对偶的复合体为复合大截半二十面体大菱形三十面体。其共有62个面、120条边和62个顶点,其尤拉示性数为4,亏格为-1,具有12个非凸面,在威佐夫记号中以(2 | 5/2 3)表示[8]。
参考文献
[编辑]- ^ Great icosidodecahedron: Related polyhedra. america.pink. [2016-08-31]. (原始内容存档于2016-08-31).
- ^ W94 Great Icosidodecahedron. colinspics. [2016-08-31]. (原始内容存档于2016-08-31).
- ^ Uniform Polyhedra 54: Great Icosidodecahedron. mathconsult. [2016-08-31]. (原始内容存档于2016-08-31).
- ^ Construction of Great IcosiDodecahedron (PDF). network solutions. [2016-08-31]. (原始内容存档 (PDF)于2016-08-31).
- ^ Gid (Great Icosidodecahedron)-Facetings. polyedergarten. [2016-08-31]. (原始内容存档于2016-03-23).
- ^ Weisstein, Eric W. (编). Great Icosidodecahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Smith, A.G. Cut and Assemble 3-D Star Shapes. Dover Children's Activity Bks. Dover Publications, Incorporated. 1997: p. 23 [2016-08-31]. ISBN 9780486296517. (原始内容存档于2016-09-11).
- ^ compound of great icosidodecahedron and great rhombic triacontahedron. bulatov.org. [2016-08-31]. (原始内容存档于2015-09-06).