正扭歪无限面体
在几何学中,正扭歪[1][2]无限面体(英语:Regular skew apeirohedron),又称扭歪正多面体(日语:ねじれ正多面体)[注 1]是一种顶点并非全部共面的正无限面体,即每个面都全等、每个角也相等的扭歪无限面体。通常扭歪无限面体会具有正扭歪的面或扭歪的顶点图。
历史
[编辑]关于考克斯特,1926年时,约翰·弗林德斯·皮特里将扭歪多边形(非平面多边形)的概念推广到四维空间的扭歪多面体和三维空间的扭歪无限面体。
考克斯特找到了三种形式,他们具有平的面和扭歪的顶点图,两者彼此互补。它们都可以用施莱夫利符号的扩展符号{l,m|n}来表示。这个扩展符号{l,m|n}表示每个顶点都是个正边形的公共顶点,且存在正边形的空洞。
若一扭歪无限面体是一个正扭歪无限面体,则其施莱夫利符号存在下列等式:
- 2 sin(π/l) · sin(π/m) = cos(π/n)
三维空间的正扭歪无限面体
[编辑]三维空间中有三种扭歪无限面体,分别为四角六片四角孔扭歪无限面体、六角四片四角孔扭歪无限面体和六角六片三角孔扭歪无限面体。约翰·康威将他们称为多立方体(英语:Mucube)、多八面体(英语:Muoctahedron)和、多四面体(英语:Mutetrahedron),英文中的字首mu-表示“多”(英语:multiple)的意思,其意义分别代表“很多立方体”、“很多八面体”以及“很多四面体”[3]。
- 四角六片四角孔扭歪无限面体(多立方体、英语:Mucube):{4,6|4}:每个顶点都是六个正方形的公共顶点
- 六角四片四角孔扭歪无限面体(多八面体、英语:Muoctahedron):{6,4|4}:每个顶点都是四个六边形的公共顶点
- 六角六片三角孔扭歪无限面体(多四面体、英语:Mutetrahedron):{6,6|3}:每个顶点都是六个六边形的公共顶点
考克斯特给予这些 {2q,2r|p} 形式的扭歪无限面体与抽象群 (2q,2r|2,p) 同构的[[(p,q,p,r)]+的手征对称性。与之相关的堆砌就具有[[(p,q,p,r)]]的扩展对称性[4]。
| 考克斯特群 对称性 |
无限面体 {p,q|l} |
图像 | 面 {p} |
洞 {l} |
顶点图 | 相关堆砌 | |
|---|---|---|---|---|---|---|---|
[[4,3,4]] [[4,3,4]+] |
{4,6|4} 四角六片四角孔 扭歪无限面体 多立方体 |
 动画 |
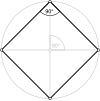 |
 |
扭歪六边形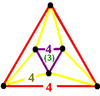 (黄色部分) |
t0,3{4,3,4} |
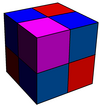
|
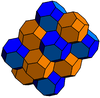
| {6,4|4} 六角四片四角孔 扭歪无限面体 多八面体 |
 动画 |
 |
扭歪四边形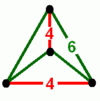 (绿色部分) |
2t{4,3,4} |
| ||
[[3[4]]] [[3[4]]+] |
{6,6|3} 六角六片三角孔 扭歪无限面体 多四面体 |
 动画 |
 |
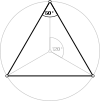 |
扭歪六边形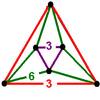 (绿色部分) |
q{4,3,4} |
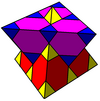
|
三维双曲空间的正扭歪无限面体
[编辑]1967年时,C. W. L. Garner以类似于在欧式三维空间寻找正扭歪无限面体的方式,发现了31种双曲空间中具有扭歪多边形顶点图的正扭歪无限面体[5]。
14种紧空间正扭歪无限面体
[编辑]| 考克斯特群 | 无限面体 {p,q|l} |
面 {p} |
洞 {l} |
堆砌 | 顶点图 | 无限面体 {p,q|l} |
面 {p} |
洞 {l} |
堆砌 | 顶点图 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
[3,5,3] |
{10,4|3} | 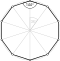 |
 |
2t{3,5,3} |
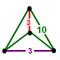 |
{4,10|3} |  |
 |
t0,3{3,5,3} |

| |
[5,3,5] |
{6,4|5} |  |
 |
2t{5,3,5} |
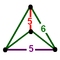 |
{4,6|5} |  |
 |
t0,3{5,3,5} |
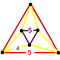
| |
[(4,3,3,3)] |
{8,6|3} |  |
 |
ct{(4,3,3,3)} |
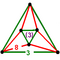 |
{6,8|3} |  |
 |
ct{(3,3,4,3)} |
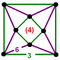
| |
[(5,3,3,3)] |
{10,6|3} |  |
 |
ct{(5,3,3,3)} |
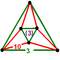 |
{6,10|3} |  |
 |
ct{(3,3,5,3)} |

| |
[(4,3,4,3)] |
{8,8|3} |  |
 |
ct{(4,3,4,3)} |
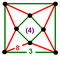 |
{6,6|4} |  |
 |
ct{(3,4,3,4)} |
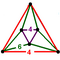
| |
[(5,3,4,3)] |
{8,10|3} |  |
 |
ct{(4,3,5,3)} |
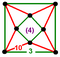 |
{10,8|3} |  |
 |
ct{(5,3,4,3)} |
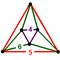
| |
[(5,3,5,3)] |
{10,10|3} |  |
 |
ct{(5,3,5,3)} |
 |
{6,6|5} |  |
 |
ct{(3,5,3,5)} |
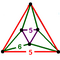
|
17种仿紧空间正扭歪无限面体
[编辑]| 考克斯特群 | 无限面体 {p,q|l} |
面 {p} |
洞 {l} |
堆砌 | 顶点图 | 无限面体 {p,q|l} |
面 {p} |
洞 {l} |
堆砌 | 顶点图 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
[4,4,4] |
{8,4|4} |  |
 |
2t{4,4,4} |
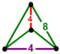 |
{4,8|4} |  |
 |
t0,3{4,4,4} |

| |
[3,6,3] |
{12,4|3} |  |
 |
2t{3,6,3} |
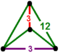 |
{4,12|3} |  |
 |
t0,3{3,6,3} |
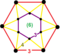
| |
[6,3,6] |
{6,4|6} |  |
 |
2t{6,3,6} |
 |
{4,6|6} |  |
 |
t0,3{6,3,6} |

| |
[(4,4,4,3)] |
{8,6|4} |  |
 |
ct{(4,4,3,4)} |
 |
{6,8|4} |  |
 |
ct{(3,4,4,4)} |

| |
[(4,4,4,4)] |
{8,8|4} |  |
 |
q{4,4,4} |

| ||||||
[(6,3,3,3)] |
{12,6|3} |  |
 |
ct{(6,3,3,3)} |
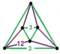 |
{6,12|3} |  |
 |
ct{(3,3,6,3)} |

| |
[(6,3,4,3)] |
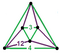
{12,8|3} |  |
 |
ct{(6,3,4,3)} |
 |
{8,12|3} |  |
 |
ct{(4,3,6,3)} |

| |
[(6,3,5,3)] |
{12,10|3} |  |
 |
ct{(6,3,5,3)} |
 |
{10,12|3} |  |
 |
ct{(5,3,6,3)} |

| |
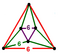
[(6,3,6,3)] |
{12,12|3} |  |
 |
ct{(6,3,6,3)} |
 |
{6,6|6} |  |
 |
ct{(3,6,3,6)} |
|
参见
[编辑]注释
[编辑]- ^ 对于此主题(无限多个面的扭歪多面体),将之称为“扭歪正多面体”可能会有歧义,因为非无限多面的扭歪多面体也可能是正多面体,例如正多面体#皮特里对偶。
参考文献
[编辑]- ^ 400年ぶりに新種の「対称性多面体」構造が発見される. gigazine.net. 2014-02-22 [2016-07-16]. (原始内容存档于2020-11-19).
- ^ 扭歪の意味. Weblio日中中日辞典. [2024-04-23]. (原始内容存档于2013-07-20).
- ^ The Symmetry of Things, 2008, Chapter 23 Objects with Primary Symmmetry, Infinite Platonic Polyhedra, pp. 333–335
- ^ Coxeter, Regular and Semi-Regular Polytopes II 2.34)
- ^ Garner, C. W. L. Regular Skew Polyhedra in Hyperbolic Three-Space. Canad. J. Math. 19, 1179–1186, 1967. [1] (页面存档备份,存于互联网档案馆) Note: His paper says there are 32, but one is self-dual, leaving 31.



